Two Trains

In my school days, I remember (and not fondly) problems like:

A train leaves Toronto for Montreal at the same time as another train leaves Montreal for Toronto. The cities are 500km apart. The trains pass each other 2h later. The train from Montreal is traveling 50km/h faster than the one from Toronto. At what distance away from Toronto do the trains pass each other?

I’m no whiz with a video camera or script writing. I’m not much of an actor (which you’ll see if you bother to watch the video below). But I think this is a more compelling way of presenting the same problem.

Act I

Act II

Act III

Answer

Sequels

I need some help here. Any ideas? Is it worth bothering?

• John was driving slower because he thought Darlene would drive farther to the meeting point. His plan is to drive 110 km/h all the way back, thinking that this would save him time overall. Would he have been better off driving 110 km/h the whole way?
• What if Darlene left an hour later?

Production Notes: My wife says that there’s no way I’d be that calm if I had to drive her purse back towards home.

Read Full Post »

Amazing Watermelons – 3 Acts

I’m starting to see this stuff more places. This one could be fun. This one is easier mathematically than the Penny Pyramid that Dan Meyer describes here. In Dan’s 3 Act Format, here it is.

Act I

Video

Ask the kids what they wonder about. They could go lots of interesting directions with this one.

Act II

Video

This video will give them the information they need, assuming their perplexing questions require them to know how many watermelons are in the pyramid.

Act III

The Answer

The answer is in the form of a Word document with a photo and some calculations. I’d love a video. If anyone has the budget for 385 watermelons, the patience to stack them, and the video editing skills to insert a counter to the filmed stacking, I’d gladly take it.

Sequels

What is the mass of the pyramid?

What is the cost of the pyramid?

How many watermelons could we stack in this room?

If the truck is 20 m from the pyramid, how long would it take to build?

Any other sequels? If so, throw them into the comments.

Download

This zip file (29.7 MB) contains both videos.

Read Full Post »

Graphing Inequalities

Last week, I mentioned to a group of teachers that I had never come up with a good way to teach kids where to shade when graphing an inequality. Vicky from one of our local high schools shared her method with me. It’s pretty nice.

Vicky gives her students an inequality like 

She asks them to each find two coordinates that satisfy the inequality, and then plot them on a giant grid at the front of the room. When 30 kids come up and plot points, it will look something like this.

From this graph, it becomes pretty obvious that there is a line involved, and which side of the line we should shade. It also becomes obvious that one kid made a mistake.

We could extend this method to quadratic inequalities. If the students were given the inequality  , we could ask students to find ordered pairs that satisfy the inequality, and plot them on a grid at the front. It might look like this.

Students could then have conversations about which of the shading should include the boundary, and which should not, and how to deal with that.

Read Full Post »

RAFT in Math

Several years ago, I attended a session on differentiated instruction with a wonderful woman named Dr. Vera Blake. One of the suggestions she made was that we use the RAFT model as a check for understanding. For those of us more mathematical than Englishal,  RAFT is a writing tool typically used in English classes. RAFT stands for: Role, Audience, Format, and Topic. It helps students focus their writing by clarifying what their role is, who their audience is, what format is appropriate, and what topic needs to be covered. I may have just demonstrated a rudimentary understanding of the process, but I’m a math teacher…

I asked Dr. Blake to tell me how I might use it in a Math class. She showed me how to use it as a review, and a check for understanding. I was wrapping up a quadratics unit with an11th grade class, and she helped me write a set of RAFTs to use as a review with them.  I created as many as I could think of, and had pairs of students randomly select one RAFT.  For example, one pair was given the role of the discriminant. Their audience was a quadratic function, and the format was a letter from a stalker.  The topic was “I know all about you!”  A pair of quiet and shy girls wrote a really creepy letter from the discriminant to the quadratic function. Their letter clearly demonstrated understanding of what the discriminant indicated about the graph of the corresponding function.

The class had a lot of fun with it. We had songs, raps, poems, letters, posters, radio ads and many other things performed in class after one day of preparation. Some other examples included a quadratic formula writing a cover letter to a quadratic equation to apply for a job, a dating ad written by a quadratic formula who was looking for love and understanding, and a workout plan devised by a personal trainer aimed at making a specific quadratic function skinnier. What all of them had in common, was that they showed an understanding of the class material.

There were some bumps. The group that had to write the dating ad had no idea what a dating ad looked like, so they searched personal ads on my computer. That probably wasn’t a good career move. Another group had to design a twelve step process in the manner of AA to solve a problem. They also searched on my computer for addiction programs. Despite it all, I managed to keep my job.

I had also forgotten to consider assessment, so I forced a rubric on their presentations in the end. I should have left it as a formative assessment.

If you are interested in trying one of the two I created, feel free. I’d love to hear from you about how it went.

Quadratic Equations and Functions RAFT Topics – Math 20-1 and Math 20-2

Relations and Functions RAFT Topics – Math 10C

Here are some samples of student work from the 11th grade class on quadratics. They were a little better when seen performed live, in front of the class, but you will get the idea. The girls who wrote the first letter are clearly better students of English than I am.

Read Full Post »

Making Other Radical Sets

Thanks to the comments on my previous post, I learned that there is a much easier way to make some of the sets I described.  To make the set, I originally suggested measuring a 60 degree angle.  Here is a much better way.

Use the  set that was built in the previous post, and place it on a different color of card stock grid paper.

Create a right triangle with legs 1 and .  This makes the hypotenuse .

Use the rest of the  set to make right triangles with legs 2 and , 3 and , 4 and , 5 and , 6 and , 7 and , and 8 and .

Cut them out, and you have a much easier  set to put on your radical ruler.

You could use similar patterns to create other sets.  For example, you can make a  set by using your  set.  Make triangles with legs 1 and  and their multiples, and you have a  set.

Thanks again for all the feedback on that last post.  You have helped make this activity even better.

Read Full Post »

Radical Ruler

Last year I visited Shannon Sookochoff at Victoria School.  She showed me a neat activity on simplifying radicals on a Geoboard.  As I was thinking about how to introduce adding and subtracting radicals recently, that activity came back to me. I have created an extension of what Shannon Showed me.

Adding and Subtracting Radicals Using a Radical Ruler

Have students create “sets” of triangles.  The set I would start with is the 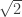 set.

Use a 1 cm grid on a piece of colored card stock.  Have students draw right triangles with legs 1 and 1 (makes the hypotenuse 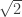) and then with legs 2 and 2 (makes the hypotenuse ) and so on.  Continue all the way to one with legs 8 and 8 (makes the hypotenuse ) .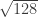

Their grid paper will look something like this:

Notice I labeled the hypotenuse inside each triangle.  That’s because we are going to cut them out so we’ll have a set that looks like this:

Next, draw a line on a piece of paper.  This will be our 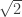 number line.  Start by putting the  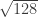triangle on the number line, and marking this point as 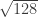.  Work your way in by putting the next one, which is , on the line.  Continue until you get down to the .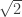

At this point, students should notice that all the gaps are 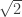 in length.

This will allow them to label the bottom of the number line with 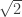, , , 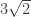and so on. Notice that this gives a nice visual showing entire radicals and their equivalent simplified mixed radical.

Now we will extend the 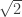 number line.  The quickest way to do this is to use the  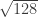triangle and place it on  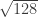as shown.

Then just mark the intersections along the hypotenuse.  These are each 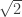 long, so we can extend our number line.  I took mine to .

Now we can add radicals pictorially on our number line.  For example, if we wanted to calculate , just put the two triangles on the number line as illustrated, and read the mixed radical below.

The answer is .

Now you can have the students make other sets.  The easiest to make are ones that don’t require any angle measurements.  I would make a 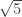 set in a different color, by using legs 1 and 2 cm long, and then just extending those by multiples of the original sides (your other legs are 2 and 4, 3 and 6, 4 and 8, 5 and 10, 6 and 12).

Cut them out, and add them to a second number line below the first. Line up the 0’s, so that students could compare and order mixed radicals using these number lines.

Other sets that are easy to make are 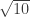 (Legs 1 and 3, and then multiples of those),  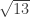(Legs 2 and 3 and then multiples of those), and  (Legs 1 and 4 and then multiples of those).  You could make as many of these as you want.

It would be nice to have a 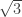 set, too, but this one will require some angle measurement.  Start with a base of 1, then measure an angle of 60 degrees. Where this meets the vertical will be a height of .

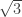Continue this with bases of 2, 3, 4, 5, 6, and 7 to create a 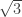 set. (Sorry about the stray line)

Cut out this set, and add it to your number line. Note that this set is much harder to build accurately.  I measured as carefully as I could, and my gaps are not consistent.

Edit: I have made improvements to the creation of the a 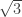 set based on feedback from the comments section below.  You can see a better way to make the a  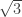set here.

Now that you’ve built these radical rulers, here are a few things you can do with them.

1. Simplifying radicals.  This is a quick pictorial representation of what radicals become in simplified form.
2. Comparing and ordering radicals.  Which is greater: or ?  Typically, students would punch this in a calculator and compare decimals. It works, but has no understanding behind it. With their radical rulers, they can see which is bigger.
3. Adding and subtracting radicals.  Put the triangles on the number line as shown above.  To illustrate adding only like radicals, ask them to add  and .  They will discover that it can’t be done.  , in green, is not like , which is yellow.

I’d love some feedback on this one.  It’s not something I have ever tried in a classroom, but I would love it if someone tries it, and lets me know how it worked.

Read Full Post »